Digitization help student to explore and study their academic courses online, as this gives them flexibility and scheduling their learning at their convenience. Kidsfront has prepared unique course material of Quantitative Aptitude Area and Volume for SBI PO Exam student. This free online Quantitative Aptitude study material for SBI PO Exam will help students in learning and doing practice on Area and Volume topic of SBI PO Exam Quantitative Aptitude. The study material on Area and Volume, help SBI PO Exam Quantitative Aptitude students to learn every aspect of Area and Volume and prepare themselves for exams by doing online test exercise for Area and Volume, as their study progresses in class. Kidsfront provide unique pattern of learning Quantitative Aptitude with free online comprehensive study material and loads of SBI PO Exam Quantitative Aptitude Area and Volume exercise prepared by the highly professionals team. Students can understand Area and Volume concept easily and consolidate their learning by doing practice test on Area and Volume regularly till they excel in Quantitative Aptitude Area and Volume.
 If the volume of a sphere is numerically equal to its surface area then its diameter is:
If the volume of a sphere is numerically equal to its surface area then its diameter is: Correct Answer Is : 6cm
Correct Answer Is : 6cm 5 person will live in a tent. If each person requires 16m2 of floor area and 100m3 space for air then the height of the cone of smallest size to accommodate these persons would be?
5 person will live in a tent. If each person requires 16m2 of floor area and 100m3 space for air then the height of the cone of smallest size to accommodate these persons would be? Correct Answer Is : 18.75m
Correct Answer Is : 18.75m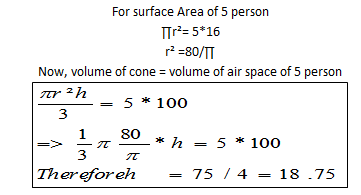
 If the altitude of an equilateral triangle is 12√3cm, then its area would be:
If the altitude of an equilateral triangle is 12√3cm, then its area would be: Correct Answer Is : 144√3cm2
Correct Answer Is : 144√3cm2
 The area of the triangle formed by the graphs of the equations x=0, 2x+3y=6 and x+y=3 is:
The area of the triangle formed by the graphs of the equations x=0, 2x+3y=6 and x+y=3 is: Correct Answer Is : 1 1/2sq.unit
Correct Answer Is : 1 1/2sq.unit
 If D, E and F are the mid points of BC, CA and AB respectively of the AABC then the ratio of area of the parallelogram DEFB and area of the trapezium CAFD is :
If D, E and F are the mid points of BC, CA and AB respectively of the AABC then the ratio of area of the parallelogram DEFB and area of the trapezium CAFD is : Correct Answer Is : 2:3
Correct Answer Is : 2:3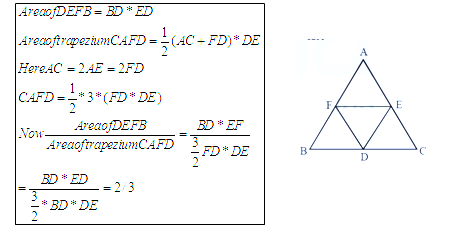
 Average weight of 3 men, A, B, C is 84 kg. Another man D joins the group and the
average now becomes 80 kg. If another man E whose weight is 3 kg more than that of D replaces A then the average weight of B, C, D and E becomes 79 kg. The weight of A in kg. is
Average weight of 3 men, A, B, C is 84 kg. Another man D joins the group and the
average now becomes 80 kg. If another man E whose weight is 3 kg more than that of D replaces A then the average weight of B, C, D and E becomes 79 kg. The weight of A in kg. is Correct Answer Is : 75
Correct Answer Is : 75 The area of the triangle formed by the graphs of the equations x = 4, y = 3 and 3x+ 4y = 12 is
The area of the triangle formed by the graphs of the equations x = 4, y = 3 and 3x+ 4y = 12 is Correct Answer Is : 6 sq. unit
Correct Answer Is : 6 sq. unit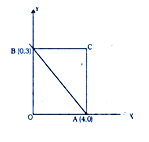
 If the area of the base, height and volume of a right prism be ((3√3)/2) P2 cm2,100√3 cm and 7200 cm’ ‘respectively, then the value of P will be
If the area of the base, height and volume of a right prism be ((3√3)/2) P2 cm2,100√3 cm and 7200 cm’ ‘respectively, then the value of P will be
 Correct Answer Is : 4
Correct Answer Is : 4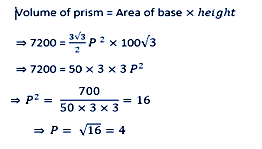
 AB and CD are two parallel chords of a circle lying on the opposite side of the
centre and the distance between them is 17 cm. The length of AB and CD are 10 cm and 24 cm respectively. The radius (in cm) of the circle is :
AB and CD are two parallel chords of a circle lying on the opposite side of the
centre and the distance between them is 17 cm. The length of AB and CD are 10 cm and 24 cm respectively. The radius (in cm) of the circle is :
 Correct Answer Is : 13
Correct Answer Is : 13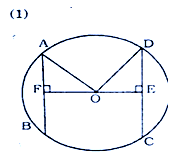
 AC is transverse common tangent to two circles with centres P and Q and radii 6
cm and 3 cm at the point A and C respectively. If AC cuts PQ at the point B and AB = 8cm then the length of PQ is :
AC is transverse common tangent to two circles with centres P and Q and radii 6
cm and 3 cm at the point A and C respectively. If AC cuts PQ at the point B and AB = 8cm then the length of PQ is :
 Correct Answer Is : 15, cm
Correct Answer Is : 15, cm